Decoding the rhythms of the natural world
Patterns are everywhere in nature, from seasons to colour schemes to heartbeats. The more you look, the more you find, and the more complex they become. Dr Bard Ermentrout of the University of Pittsburgh uses advanced mathematics to decipher these patterns
The natural world is a complex place and being able to understand it requires complex tools. Dr Bard Ermentrout, Distinguished University Professor at the University of Pittsburgh in the USA, has plenty of expertise in applying these tools and learning exactly what the natural world has to tell us. Two of his recent projects involve understanding ‘flicker hallucinations’ and the mechanisms behind seashell colouration patterns.
FLICKERS OF UNDERSTANDING
Have you ever noticed how geometric shapes can appear in your field of vision when you’re looking at flickering light? These ‘flicker hallucinations’ form an illusory pattern in your visual field, and Bard has undertaken research to understand exactly how and why this takes place. “Maths provides a framework for understanding many natural phenomena, and very different instances can lead to similar mathematical patterns,” he says.
In a very different scenario, if you add oil to a warming frying pan and then evenly add pepper or paprika, the spice will separate into distinct geometric structures. Mathematical biologists call this ‘spontaneous symmetry breaking’. It is the same general process that happens when the light uniformly hitting our eyes appears to break into distinct geometric shapes. “In terms of physics, there are big differences, but it’s the same underlying mathematics,” says Bard.
These processes occur when something ‘loses stability’; it changes from a normal state (like the everyday function of our eyes) into something else. You can achieve this effect with all kinds of things. For instance, if moved, a pendulum will swing back and forth until it comes to rest. However, if you move the base of the pendulum a certain way at the same time, it will give the appearance of going haywire. It is the same principle as swinging your legs back and forth to go higher on a swing. Using mathematics to understand these unstable states when they occur in biology can reveal interesting secrets.
BREAKING IT DOWN
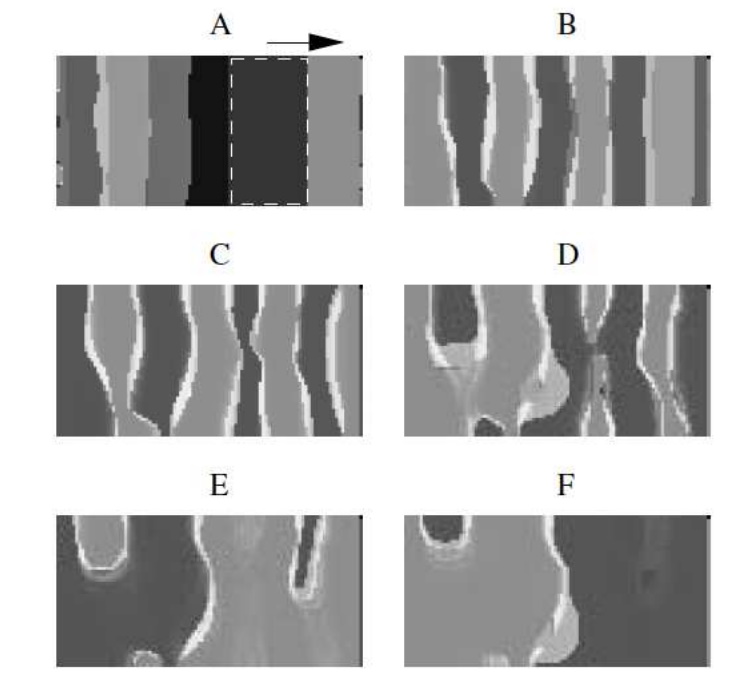
Studying flicker hallucinations can be challenging because the shapes are difficult to control and report. Bard’s team managed to simplify the effect by reducing the flickering light to a thin ring. They were then able to quantify the effects they saw, ending up with data that could be mathematically processed.
Psychologists were also brought in to understand certain phenomena. “We noticed that the patterns on the ring of flickering light appeared to rotate, first one way and then the other,” says Bard. Understanding how the brain processes visual information is critical to uncovering why these odd effects are seen.
So, what’s the purpose of this research? One theory is that these shapes provide a window into early visual systems, in terms of in the first processing units in the cortex (the main part of our brains). These systems would interpret light or darkness as a simple ‘on or off’, which is perhaps why certain flickering lights appear to us as block geometric shapes. The patterns that we see are directly related to the way that the neurons are connected in this part of the cortex. Bard explains, “Given the connectivity, we can infer the patterns and, similarly, given the patterns, it is sometimes possible to infer the connectivity. As a famous example, if you put powder on top of a drum head and then you bow it on the side to cause a vibrating sound, there will be a pattern on the drum head that is directly related to both the shape of the drum and the tone of the vibration.”
Experimentalists see many oscillations in the brain and these come in the form of waves that have been shown to reflect the connectivity between regions of the brain. “The patterns that we describe are at a much smaller scale, but follow the same principles,” says Bard. Using mathematics to model these effects can build our understanding of not only human perception but also that of other organisms, present and past.
THE SCIENCE OF SEASHELLS
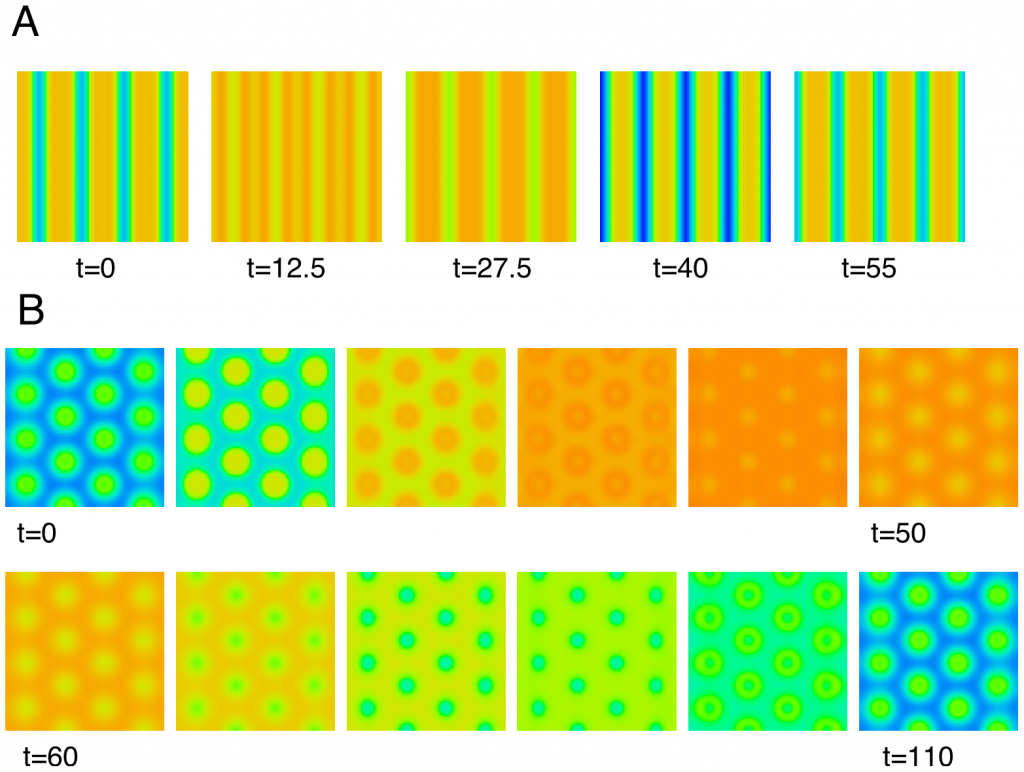
The principles of mathematical biology can be applied far and wide. Bard has worked with an evolutionary biologist to understand the complex patterns found on cone shells, a visually striking (and very venomous) family of marine molluscs. They found spontaneous symmetry breaking was the culprit again. “Uniform pigmentation became unstable, leading to stripes and other intricate patterns on the shells,” says Bard.
The biological stimulus for this effect came from the mollusc’s nervous system. As the shell grows, a simple neural network retains a ‘memory’ of the previous pattern and tells the pigment-producing cells to do the same again.
Ultimately, this research led to a better understanding of cone shells’ evolutionary history, and even helped evolutionary biologists predict what the shells’ ancestors would have looked like millions of years ago.
A MODERN SCENARIO
Mathematical biology and an understanding of patterns can also be used to tackle pressing modern issues. The COVID-19 pandemic is a prime example. Epidemiology involves complex maths to understand how viruses spread from one person to another, the roles of people without symptoms (‘super-spreaders’), how the virus exists outside of the body and so on. Bard explains, “The spread of the virus depends on who the infected are connected to, so as with the brain patterns, the spread of the disease is directly related to who is connected to whom. The patterns reflect the underlying network.” On a micro level, maths helps build models of the human immune system and the virus within it, which could help answer why some people are very deeply affected while others may not even know they are infected. Finding these answers is critical to saving lives.
TALK LIKE A MATHEMATICAL BIOLOGIST
EPIDEMIOLOGY
The study of how diseases spread and can be controlled.
LINEAR MODEL
A model is linear if changes in the inputs result in proportional outputs of the model.
NON-LINEAR MODEL
A mathematical model that does not follow a straight line on a graph. A model is non-linear if it is not linear!
PHENOMENA
Something observed, like a situation or event.
SPATIAL
Related to space.
TEMPORAL
Related to time.
Reference
https://doi.org/10.33424/FUTURUM91
Simulations of the pigmentation patterns on seashells. The model is based on the idea that the nervous system of the mollusc is able to detect the history of the pigment that has been previously deposited to determine what pattern to secrete next. The panel labelled B shows the simulation before projecting it onto an appropriately shaped shell.
If a person’s eyeball is directly stimulated with an alternating current and a black bar is moved across the field of view (dashed box in panel A), then there is an illusion of slowly moving contours that merge and reform (panels B-F).
FLICKERS OF UNDERSTANDING
Have you ever noticed how geometric shapes can appear in your field of vision when you’re looking at flickering light? These ‘flicker hallucinations’ form an illusory pattern in your visual field, and Bard has undertaken research to understand exactly how and why this takes place. “Maths provides a framework for understanding many natural phenomena, and very different instances can lead to similar mathematical patterns,” he says.
In a very different scenario, if you add oil to a warming frying pan and then evenly add pepper or paprika, the spice will separate into distinct geometric structures. Mathematical biologists call this ‘spontaneous symmetry breaking’. It is the same general process that happens when the light uniformly hitting our eyes appears to break into distinct geometric shapes. “In terms of physics, there are big differences, but it’s the same underlying mathematics,” says Bard.
These processes occur when something ‘loses stability’; it changes from a normal state (like the everyday function of our eyes) into something else. You can achieve this effect with all kinds of things. For instance, if moved, a pendulum will swing back and forth until it comes to rest. However, if you move the base of the pendulum a certain way at the same time, it will give the appearance of going haywire. It is the same principle as swinging your legs back and forth to go higher on a swing. Using mathematics to understand these unstable states when they occur in biology can reveal interesting secrets.
BREAKING IT DOWN
Studying flicker hallucinations can be challenging because the shapes are difficult to control and report. Bard’s team managed to simplify the effect by reducing the flickering light to a thin ring. They were then able to quantify the effects they saw, ending up with data that could be mathematically processed.
Psychologists were also brought in to understand certain phenomena. “We noticed that the patterns on the ring of flickering light appeared to rotate, first one way and then the other,” says Bard. Understanding how the brain processes visual information is critical to uncovering why these odd effects are seen.
So, what’s the purpose of this research? One theory is that these shapes provide a window into early visual systems, in terms of in the first processing units in the cortex (the main part of our brains). These systems would interpret light or darkness as a simple ‘on or off’, which is perhaps why certain flickering lights appear to us as block geometric shapes. The patterns that we see are directly related to the way that the neurons are connected in this part of the cortex. Bard explains, “Given the connectivity, we can infer the patterns and, similarly, given the patterns, it is sometimes possible to infer the connectivity. As a famous example, if you put powder on top of a drum head and then you bow it on the side to cause a vibrating sound, there will be a pattern on the drum head that is directly related to both the shape of the drum and the tone of the vibration.”
Experimentalists see many oscillations in the brain and these come in the form of waves that have been shown to reflect the connectivity between regions of the brain. “The patterns that we describe are at a much smaller scale, but follow the same principles,” says Bard. Using mathematics to model these effects can build our understanding of not only human perception but also that of other organisms, present and past.
THE SCIENCE OF SEASHELLS
The principles of mathematical biology can be applied far and wide. Bard has worked with an evolutionary biologist to understand the complex patterns found on cone shells, a visually striking (and very venomous) family of marine molluscs. They found spontaneous symmetry breaking was the culprit again. “Uniform pigmentation became unstable, leading to stripes and other intricate patterns on the shells,” says Bard.
The biological stimulus for this effect came from the mollusc’s nervous system. As the shell grows, a simple neural network retains a ‘memory’ of the previous pattern and tells the pigment-producing cells to do the same again.
Ultimately, this research led to a better understanding of cone shells’ evolutionary history, and even helped evolutionary biologists predict what the shells’ ancestors would have looked like millions of years ago.
A MODERN SCENARIO
Mathematical biology and an understanding of patterns can also be used to tackle pressing modern issues. The COVID-19 pandemic is a prime example. Epidemiology involves complex maths to understand how viruses spread from one person to another, the roles of people without symptoms (‘super-spreaders’), how the virus exists outside of the body and so on. Bard explains, “The spread of the virus depends on who the infected are connected to, so as with the brain patterns, the spread of the disease is directly related to who is connected to whom. The patterns reflect the underlying network.” On a micro level, maths helps build models of the human immune system and the virus within it, which could help answer why some people are very deeply affected while others may not even know they are infected. Finding these answers is critical to saving lives.
TALK LIKE A MATHEMATICAL BIOLOGIST
EPIDEMIOLOGY
The study of how diseases spread and can be controlled.
LINEAR MODEL
A model is linear if changes in the inputs result in proportional outputs of the model.
NON-LINEAR MODEL
A mathematical model that does not follow a straight line on a graph. A model is non-linear if it is not linear!
PHENOMENA
Something observed, like a situation or event.
SPATIAL
Related to space.
TEMPORAL
Related to time.
 Dr Bard Ermentrout
Dr Bard Ermentrout
Department of Mathematics
University of Pittsburgh, USA
FIELD OF RESEARCH: Mathematical Biology
RESEARCH PROJECT: Using mathematical models to uncover patterns in nature, such as visual hallucinations or pigments in mollusc shells.
ABOUT MATHEMATICAL BIOLOGY
Mathematical biology involves the construction of mathematical models to answer biological
questions. To take a simple example, say that we know a crocodile increases in length by x cm
every year (they continue growing throughout their lives, after all). How do we predict how long it will be in several years’ time? This involves constructing a formula, in this case l = xy, where l is length and y is the number of years since it was born.
This formula is a very simple model of linear dynamics. If you drew it on a graph of length against time, it would be a straight line. Most biological processes are far more complex than that, especially when it comes to patterns in nature. Bard specialises in non-linear dynamics. “Non-linear dynamics are much more complicated because there are many possible states and paths, depending on where you start,” he says. On the crocodile example, he expands, “As the crocodile grows in length, his girth also grows and, in fact, grows in a way that is proportional to his length; if his length doubles, his girth doubles. On the other hand, his weight will increase non-linearly, basically like his length cubed (since volume is proportional to length^3).”
DETECTING A PATTERN
Nature is full of patterns. Bard is interested in patterns that vary over space (such as spots or stripes) or time (such as rhythm). When we think of patterns, we most naturally think of spatial patterns, of which there are plenty of examples in the animal kingdom. Patterns that mimic an animal’s surroundings, such as a tiger’s stripes, help with camouflage, while patterns that stand out often act as a warning of danger. Recognition of these patterns can be deeply encoded within DNA. For instance, some birds will instinctively be afraid of a colourful snake, even if they have never seen a snake before in their life.
Temporal patterns are those that play out over time. For instance, think of a horse. When it is walking slowly, it moves one leg at a time; when trotting it moves diagonally opposite legs at once; and at a gallop, only one hoof touches the ground at a time. These are temporal patterns. “Gaits must be able to deal with uneven surfaces and obstacles, and using set patterns helps to overcome these,” says Bard. We have our very own temporal patterns for walking, running, cycling, rowing and any other repetitive activity that our bodies undertake.
MATHS ON (AND IN) THE BRAIN
Bard takes a special interest in applying his expertise to neuroscience. “Many neuroscientists are interested in mechanisms, perhaps more so than most other biologists,” he says. “We have new experimental methods that allow us to tackle problems that would have been unimaginable before. And, of course, the brain and nervous system are just very cool.”
The human nervous system can be affected by patterns in odd ways. “When looking at a flashing light (a temporal pattern), some people can experience migraines or even seizures,” says Bard. “In most, we will see geometric patterns called flicker phosphenes.” Optical illusions provide another example: for instance, we might see flashing dots in high-contrast patterns, when no dots are actually there. Building models of why these effects happen is one of Bard’s areas of interest.
THE MAKINGS OF A MATHEMATICIAN
“My aim is to provide explanations or mechanisms for things we observe in the natural world,” says Bard. Being able to do this requires a comprehensive knowledge of modelling, and also the tools to put these into practice. “You need to choose the right tool, and the tools available are always expanding,” he says. Models involve a simplification of a complex, messy system, and making sure that you simplify in the right direction is crucial. As Einstein said, “A model should be as simple as it can be but no simpler.” Collaboration is also vitally important. Biologists tend not to have an in-depth knowledge of mathematics, and vice versa. Combining unique sets of skills is a cornerstone of good science.
• There are some undergraduate degrees available in mathematical biology, but more common degrees such as mathematics and, sometimes, biology can also lead to a career in this area.
• According to The Complete University Guide, the top UK universities for mathematics are Oxford, Cambridge, St Andrews, Durham and Imperial College London.
• According to Top Universities, the top US universities for mathematics are MIT, Harvard, Stanford, California Berkeley and Princeton. Bard advises that the best universities for mathematical biology are less well-known and include Utah, Michigan, Columbia and New York University.
PATHWAY FROM SCHOOL TO MATHEMATICAL BIOLOGIST
At school, mathematics, further mathematics, biology and other sciences are advantageous. At university, Bard recommends taking classes in calculus and linear algebra, and, if possible, basic science, probability and statistics, and differential equations.
Programming and coding ability will also prove useful. Bard advises learning LaTeX, the digital tool used for writing mathematical formulae and models, as well as some sort of higher-level programming language like MatLab or Python.
HOW DID DR BARD ERMENTROUT BECOME A MATHEMATICAL BIOLOGIST?
WHAT WERE YOUR INTERESTS AS A CHILD?
From an early age, I was very interested in science and knew I wanted to be a scientist. I collected insects and performed lots of chemistry experiments. I would make cultures of different bacteria and then use my microscope and staining techniques to try to identify them. I was very good at maths in high school and considered becoming a doctor before I settled on science.
WHAT INSPIRED YOUR CAREER?
At university, I began studying chemistry but quickly switched to pure maths after an advanced chemistry class introduced me to group theory. In my last year, I switched gears completely, when my attention was grabbed by a class on maths applied to biology. My interest was sparked further by a book on theoretical biology that covered catastrophe theory and neural networks. This led me to apply to the Department of Theoretical Biology at the University of Chicago. There, I was able to pursue my interests in applications of maths to neuroscience.
WHAT HAVE BEEN YOUR PROUDEST ACHIEVEMENTS SO FAR?
I co-developed the theory of weakly coupled oscillators, which I continue to build upon. I also built a software platform for modelling differential equations that is used by students and researchers around the world. I am also proud of the many successful students whom I have mentored over three decades of teaching.
HOW DO YOU ‘SWITCH OFF’ FROM YOUR ACADEMIC WORK?
I am an avid cook. My wife and I have a very well-equipped kitchen and make almost all our meals from scratch. I make fruit ciders and have learned to make a lot of different cocktails. I also have a big vegetable garden. I start all my plants in the late winter from seed and then fight off deer, drought and fungus during the summer!
WHAT ARE YOUR FUTURE AMBITIONS?
I would like to uncover more about the reasons for the patterns we observe. For instance, why are there so many waves and oscillations happening in the brain? How do they relate to higher brain function?
BARD’S TOP TIPS FOR STUDENTS
1. Find something that truly excites you and follow it.
2. Learn how to communicate with other scientists, especially those who might not know much mathematics.
3. Find some good mentors and, if you can, work on projects alongside them.
Do you have a question for Bard?
Write it in the comments box below and Bard will get back to you. (Remember, researchers are very busy people, so you may have to wait a few days.)